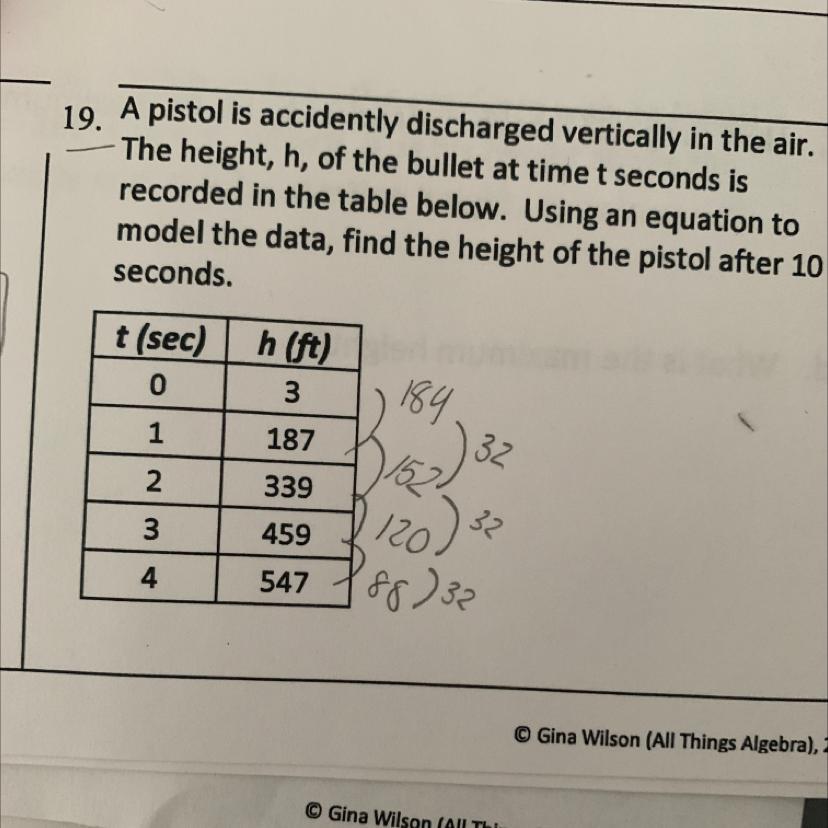
A pistol is accidently discharged vertically in the air. The height, h, of the bullet at time t seconds is recorded in the table below. Using an equation to model the data, find the height of the pistol after 10 seconds.

t (sec)
0
1
2
3
4
h (ft)
3
187
339
459
547

The critical value of the chi-square distribution corresponding to a sample size of 5 and a confidence level of 98% is given as follows:

0.297 and 13.277.

To obtain a critical value, we need three parameters, given as follows:

Then, with the parameters, the critical value is found using a calculator.

The parameters for this problem are given as follows:

Using a chi-square distribution calculator, the critical values are given as follows:

0.297 and 13.277.

More can be learned about the chi-square distribution at https://brainly.com/question/4543358

#SPJ1

The correct statement is that a fairly strong negative linear relationship exists between the x and y variables.

The correlation coefficient is a measure of the strength and direction of the linear relationship between two variables. It ranges from -1 to 1, where a value of -1 indicates a perfect negative correlation, 0 indicates no correlation, and 1 indicates a perfect positive correlation.

In this case, the correlation coefficient is -0.88, which indicates a strong negative linear relationship between the x and y variables. This means that as the value of x increases, the value of y decreases in a predictable manner.

The negative sign of the correlation coefficient indicates that the relationship is negative, meaning that as one variable increases, the other variable tends to decrease. The absolute value of the correlation coefficient, 0.88, indicates a strong relationship, meaning that the values of the two variables are closely related and can be used to predict each other's values.

Therefore, the correct statement is that a fairly strong negative linear relationship exists between the x and y variables.

Learn more about correlation coefficient

brainly.com/question/15577278

#SPJ11

Answer: 4080

Step-by-step explanation:

First you have to find the area of the triangle. 24*10 = 240. 240/2 = 120. Then you multiply the area of the triangle and multiply it by 34. 120 * 34 = 4080. This means the answer is 4080

Key difference between binomial distribution and hypergeometric distribution is that with hypergeometric distribution is given by

Option b. the probability of success changes from trial to trial.

In the binomial distribution, each trial is independent of the others.

Probability of success remains constant from trial to trial.

Hypergeometric distribution involves sampling without replacement.

Probability of success changes from trial to trial as the size of the population changes.

Example of drawing cards from a deck.

In the binomial distribution,

if we wanted to know the probability of drawing three hearts in a row.

Probability of drawing a heart would remain constant at 13/52 .

In the hypergeometric distribution,

Probability of drawing three hearts without replacement from a deck of 52 cards.

Probability of drawing a heart would change with each trial.

As the size of the population would be different for each trial.

The other options listed are not correct,

The trials in the hypergeometric distribution are not independent of each other.

Because sampling without replacement affects the probability of success in subsequent trials.

Hypergeometric distribution is a discrete distribution not a continuous one.

Probability of success in the hypergeometric distribution does not have to be less than 0.5.

Learn more about binomial distribution here

brainly.com/question/14210034

#SPJ4

The probability that the golfer will hit at least 6 times in his next 10 attempts is A. 20 %

To estimate the probability of the golfer hitting at least 6 times in his next 10 attempts using a table of random numbers, we can perform a simulation.

Let's use the given table of random numbers to simulate 10 attempts for each trial. We can consider each pair of digits as one attempt. We will perform 10 trials and count how many times the golfer hits at least 6 times in 10 attempts.

Now count the number of trials with at least 6 hits:

Trial 2, Trial 5, and Trial 9 have at least 6 hits. That's 3 out of 10 trials.

To estimate the probability, divide the number of successful trials (at least 6 hits) by the total number of trials:

Probability = (Number of successful trials) / (Total number of trials)

Probability = 3 / 10 = 0.3

The estimated probability that the golfer will hit at least 6 times in his next 10 attempts is 30%. There is no exact match among the possible answers, but the closest one is 20%.

Find out more on probability at https://brainly.com/question/30618573

#SPJ1

For the given numbers, 78 < 0. 708

To compare two numbers, we need to look at their values and determine which one is larger or smaller. In this case, we have 78 and 0.708. We can start by comparing their whole number parts, which are 78 and 0, respectively. Since 78 is greater than 0, we know that 78 is a larger number.

But what about the decimal parts of these numbers? To compare them, we need to look at the place value of each digit. The first digit after the decimal point in 78 is 0, and the first digit after the decimal point in 0.708 is 7. Since 7 is greater than 0, we know that 0.708 is a larger number than 0.78 in terms of their decimal parts.

Now that we have compared the whole number parts and decimal parts separately, we can combine the results to determine the final comparison. Since 78 is larger than 0 and 0.708 is larger than 0.78 in terms of their decimal parts, we can conclude that:

78 < 0.708

We use the symbol "<" here because 78 is smaller than 0.708.

To know more about number here

https://brainly.com/question/17429689

#SPJ4

The blue base is the face (put in 1).

The black line is the edge (put in 2).

The dot up top is the vertex (put in 3).

Answer:

Step-by-step explanation:

You want to know if the fractions (2 1/2)/(6.5) and (5/8)/(1 5/8) are in a proportional relationship, and the simplest form of each.

Equivalent fractions can be found by multiplying numerator and denominator by the same number.

  (2 1/2)/(6.5) = 2·(2 1/2)/(2·6.5) = 5/13

  (5/8)/(1 5/8) = 8(5/8)/(8·(1 5/8)) = 5/(8+5) = 5/13

Both fractions are equivalent to 5/13, so their relationship is proportional.

Answer:

x = -5, x = 1

As (x, y) coordinates, the x-intercepts are (-5, 0) and (1, 0).

Step-by-step explanation:

The x-intercepts are the x-values of the points at which the curve crosses the x-axis, so when y = 0.

From inspection of the given graph, we can see that the parabola crosses the x-axis at x = -5 and x = 1.

Therefore, the x-intercepts of the parabola are:

As (x, y) coordinates, the x-intercepts are (-5, 0) and (1, 0).

The ball that weighs 30g is ball A.

The degree to which a measured value resembles the true or recognised value is known as accuracy. On the other hand, precision describes how closely two measurements of the same quantity agree. In other words, precision is a measure of consistency, whereas accuracy is a measure of correctness. The values obtained are consistent but not always close to the genuine value when a measurement is precise but not exact. On the other hand, a measurement might be accurate without being exact, which means that the result is close to the correct value but produces erratic or dispersed results when repeated.

From the given weights corresponding to different balls, 30 g corresponds to ball A.

Hence, the ball that weighs 30g is ball A.

Learn more about accuracy here:

https://brainly.com/question/14244630

#SPJ1

The 'footprint' of CO2 emissions for a person in 1830 would be 818,199 tons of CO2 emissions per person.

To find the 'footprint' of CO2 emissions for a person in 1830, we need to substitute the value of x = 1830 - 1800 = 30 into the given function C(x) = 0.0365 (1.758)^x.

Plugging in x = 30 into the function, we get:

C(30) = 0.0365 * (1.758)^30

Substituting this value back into the function, we get:

C(30) = 0.0365 * 22416413.1381

C(30) = 818199.079541

C(30) ≈ 818,199.08

Answered question "Scientists studying the 'footprint' of carbon dioxide (CO2) emissions attributed to the average person for each decade from 1800 to 1910 used the function C(x) = 0.0365 (1.758)*, where x is the number of decades since 1800 and C is the number of tons of CO2 emissions per person. What is the 'footprint' of CO2 emissions for a person in 1830??"

Read more about Footprint

brainly.com/question/14441911

#SPJ1

Answer:

x + y = 11

2x - y = 19

--------------

3x = 30

x = 10, so y = 1

Answer:

Step-by-step explanation:

Answer:1.25

Step-by-step explanation:

it just math

the average speed of the plane in still air is s + 30.

Let's call the average speed of the plane in still air "s" (in miles per hour).

We can use the formula:

time = distance / speed

to find the time it takes the plane to travel from Salt Lake City to Oakland with the wind and against the wind.

With the wind:

time with wind = [tex]600 / (s + 30)[/tex]

Against the wind:

time against wind =[tex]600 / (s - 30)[/tex]

time against wind > time with wind

So we can set up an inequality:

[tex]600 / (s - 30) > 600 / (s + 30)[/tex]

Multiplying both sides by [tex](s - 30)(s + 30)[/tex], we get:

[tex]600(s + 30) > 600(s - 30)[/tex]

Expanding and simplifying, we get:

[tex]600s + 18000 > 600s - 18000[/tex]

Subtracting 600s from both sides, we get:

[tex]18000 > -18000[/tex]

This inequality is true for all values of s. In other words, there are no restrictions on the value of s that would make the return trip take longer than the trip with the wind.

Therefore, we can use the average of the two speeds (with and against the wind) to find the average speed of the plane in still air:

Average speed = [tex]2s(s + 30) / (s + 30 + s - 30)[/tex]

Simplifying, we get:

Average speed = [tex]2s(s + 30) / (2s)[/tex]

Canceling the common factor of 2s, we get:

Average speed = s + 30

We know that the distance from Salt Lake City to Oakland is 600 miles, and we can use the formula:

time = distance / speed

to find the time it takes the plane to travel this distance:

time = [tex]600 / (s + 30)[/tex]

We also know that the return trip (against the wind) takes longer, so we can set up another equation:

time return trip =[tex]600 / (s - 30)[/tex]

We can use these two equations to solve for s:

[tex]600 / (s + 30) = 600 / (s - 30)[/tex]

Cross-multiplying, we get:

[tex]600(s - 30) = 600(s + 30)[/tex]

Expanding and simplifying, we get:

[tex]600s - 18000 = 600s + 18000[/tex]

Subtracting 600s from both sides, we get:

[tex]-18000 = 18000[/tex]

This is not a valid equation, so there must be no solution.

However, we can still find the average speed of the plane in still air by using the equation we derived earlier:

Average speed = s + 30

So the average speed of the plane in still air is s + 30. We don't have a specific value for s, but we can say that the average speed is equal to the speed with the wind plus 30 (which is the speed of the wind).

for such more questions on average speed

https://brainly.com/question/4931057

#SPJ11

D) 81 is equivalent to 27^(4/3).

The expression 27^4/3 can be simplified using the rule that (a^m)^n = a^(m*n). Therefore, we can write,

27^(4/3) = (3^3)^(4/3)

Using the power of a power rule, we can simplify further,

(3^3)^(4/3) = 3^(3*4/3)

Simplifying the exponent, we get,

3^(4)

To check the other answer choices,

A. 4^3 is not equivalent to 27^4/3.

B. (27^1/3)^4 is equivalent to 27^(4/3), which we already simplified to 3^4. Therefore, this expression is also equivalent to 3^4.

C. 3^1/4 is not equivalent to 27^4/3.

D. 81 is equivalent to 3^(4).

Therefore, the expression 27^4/3 is equivalent to 3^4, which is answer choice D) 81.

To learn more about equivalent here:

https://brainly.com/question/31532746

#SPJ4

The length from point A to point B on the clock is approximately 24.083 cm, which is closest to 24 cm. This is calculated using the Pythagorean theorem.

Using the Pythagorean theorem, we can calculate the length from point A to point B as follows

First, we need to find the length of the vertical line segment from point D to point A. This is equal to the radius of the clock, which is 24 cm.

Next, we can find the length of the horizontal line segment from point D to point B. This is equal to the distance from the top of the clock at point D to the hanger at point B, which is given as 2 cm.

Now, we can use the Pythagorean theorem to find the length from point A to point B

AB² = AD² + DB²

AB² = (24 cm)² + (2 cm)²

AB² = 576 cm² + 4 cm²

AB² = 580 cm²

AB ≈ 24.083 cm

Therefore, the length from point A to point B is approximately 24.083 cm, which is closest to 24 cm.

To know more about Pythagorean theorem:

https://brainly.com/question/14930619

#SPJ1

Answer:

The length from point A to point B on the clock is approximately 24.083 cm, which is closest to 24 cm. This is calculated using the Pythagorean theorem.

Hope this helps :)

Pls brainliest...

Answer:

Step-by-step explanation:

Change in Y/Change in X

5- -1/6- -2

5+1/6+2

6/8

3/4

The only statement that is true is b, which states that the quadrilateral is a rectangle.

A quadrilateral is a polygon with four sides and four vertices. The sum of the interior angles of a quadrilateral is always 360 degrees. Quadrilaterals can have sides of different lengths and angles of different measures, giving rise to many different types of quadrilaterals with different properties.

First, we find the lengths of the sides of the quadrilateral:

AB = √[(7-6)² + (-1+4)²] = √10

BC = √[(4-7)² + (0-0)²] = 3

CD = √[(3-4)² + (-3+0)²] = √10

AD = √[(6-3)² + (-4+1)²] = √26

Then, we find the slopes of each pair of opposite sides:

AB: (7-6)/(−1+4) = 1/3

BC: (4-0)/(0-(-1)) = 4/1 = 4

CD: (-3-(-4))/(0-(-3)) = 1/3

AD: (6-3)/(-4-(-1)) = -1/5

Now we can analyze each statement:

a.) Rhombus

A rhombus is a quadrilateral with all sides of equal length. We found that AB = CD and AD ≠ BC, so not all sides are of equal length. Therefore, statement a is false.

b.) Rectangle

A rectangle is a quadrilateral with all angles equal to 90 degrees. We can find the slopes of adjacent sides and check if they are opposite reciprocals:

AB: 1/3

BC: 4

CD: 1/3

AD: -1/5

We can see that AB and CD have slopes of 1/3 and are opposite reciprocals, and BC and AD have slopes of 4 and -1/5, respectively, and are also opposite reciprocals. Therefore, all angles of the quadrilateral are 90 degrees. Also, since AB = CD and AD ≠ BC, the quadrilateral is a rectangle. Therefore, statement b is true.

c.) Square

A square is a special type of rectangle with all sides of equal length. We found that AB ≠ AD, so not all sides are of equal length. Therefore, statement c is false.

d.) None

We have determined that the quadrilateral is a rectangle, so it is not "none". Therefore, statement d is false.

Therefore, the only statement that is true is b, which states that the quadrilateral is a rectangle.

To know more about the quadrilateral and rectangle visit:

brainly.com/question/11936810

#SPJ1

The chance that the number of heads will be in the range 4850 to 5150 is approximately 0.9973, or about 99.73%.

The number of heads in 10,000 coin tosses follows a binomial distribution with parameters n = 10,000 (the number of trials) and p = 0.5 (the probability of heads on a single toss).

We can approximate this binomial distribution using the normal distribution, with mean μ = np = 5000 and variance σ² = np(1-p) = 2500.

To find the probability that the number of heads is in the range 4850 to 5150, we can use the normal distribution and standardize the range using the z-score formula:

z = (x - μ) / σ

where x is the number of heads in the range we're interested in.

For the lower bound of 4850, we have:

[tex]z_lower = (4850 - 5000) / \sqrt{(2500)}[/tex]

= -3

For the upper bound of 5150, we have:

[tex]z_upper = (5150 - 5000) / \sqrt{(2500)} = 3[/tex]

Using a standard normal distribution table or calculator, we can find the probability of being within 3 standard deviations of the mean:

P([tex]z_lower[/tex]  < Z < [tex]z_upper[/tex] ) ≈ P(-3 < Z < 3)

= 0.9973.

For similar question on distribution.

https://brainly.com/question/26678388

#SPJ11

The Cube root of the resulting number is 8.

The smallest number that 40000 can be divided by so that the result is a perfect cube, we need to factorize 40000 into its prime factors:

[tex]40000 = 2^6 \times 5^4[/tex]

To make this a perfect cube, we need to ensure that the powers of each prime factor are multiples of 3.

The smallest number we can divide 40000 by so that the result is a perfect cube is:

[tex]40000 = 2^6 \times 5^4[/tex]

Now we can find the cube root of the resulting number:

[tex]3\sqrt (40000 \div 100) = 3\sqrt400 = 8.[/tex]

Factories 40000 into its prime components in order to determine.

The least number that the result may be divided by while still producing a perfect cube.

The powers of each prime factor must be multiples of three in order for this to be a perfect cube.

The least number that 40000 may be divided by to produce a perfect cube is:

For similar questions on Cube Root

https://brainly.com/question/26726803

#SPJ11

Tim was driving for 5 hours before he caught up to Dora.

The answer is (a) 5 hours.

To solve this problem, we can use the formula:
distance = rate × time
Let's denote the time Tim drove as t hours.

Since Dora started driving one hour earlier, her driving time would be (t + 1) hours.
Dora's distance: 75 kph × (t + 1)
Tim's distance: 90 kph × t
Since Tim catches up to Dora, their distances will be equal:
75(t + 1) = 90t
Now we can solve for t:
75t + 75 = 90t
75 = 15t
t = 5.

The answer is (a) 5 hours.

For similar question on distances.

https://brainly.com/question/29657955

#SPJ11

The value of angle GFH is 18°

A circle is a special kind of ellipse in which the eccentricity is zero and the two foci are coincident.

A theorem in circle geometry starts that angle in the same segment are equal. In triangle EFG, angle F and G are on the same segment, this means that angle F and G are equal.

Represent angle F as x

therefore 144+2x = 180° ( sum of angle in a triangle)

2x = 180-144

2x = 36

x = 36/2 = 18°

Therefore the measure of angle GFH is 18°

learn more about circle geometry from

https://brainly.com/question/24375372

#SPJ1

Just use the pythagorean theorem to solve the hypotenuse!

(3^2)+(2^2)=x^2

9+4=13^2

[tex]\sqrt{13}[/tex] = [tex]\sqrt{x}[/tex]

[tex]13^{2}[/tex] km

Hope this helps <3

the simplified  form  of expression is: -(x² + 2x - 2)/((x+2)*(x+4))

what is expression  ?

In mathematics, an expression is a combination of numbers, variables, operators, and/or functions that represents a mathematical quantity or relationship. Expressions can be simple or complex

In the given question,

To evaluate the expression 1/(x+2) - (x+1)/(x+4), we need to find a common denominator for the two terms. The least common multiple of (x+2) and (x+4) is (x+2)(x+4).

So, we can rewrite the expression as:

(1*(x+4) - (x+1)(x+2))/((x+2)(x+4))

Expanding the brackets, we get:

(x+4 - x² - 3x - 2)/((x+2)*(x+4))

Simplifying the numerator, we get:

(-x² - 2x + 2)/((x+2)*(x+4))

Therefore, the simplified expression is:

-(x² + 2x - 2)/((x+2)*(x+4))

To know more about  Expressions , visit:

https://brainly.com/question/14083225

#SPJ1

Answer:

Step-by-step explanation:= x+48=180 ( linier pair )

                                            = x=180-48

                                            = x=132

                                              = x+12=180 (liner pair)

                                              = x=180-12

                                              = x=168

No, the ratio of circumference to radius is not the same for every circle.

Ratio refers to the quantitative relation between two or more values, typically expressed in the form of a fraction or a proportion.

No, the ratio of circumference to radius is not the same for every circle. The ratio of circumference to diameter, also known as pi (π), is a constant value that remains the same for every circle. It is approximately equal to 3.14 or 22/7. However, the ratio of circumference to radius varies depending on the size of the circle.

The formula for circumference of a circle is C=2πr, where C is the circumference and r is the radius. Therefore, the ratio of circumference to radius is C/r = 2π. This means that for circles of different sizes, the ratio of circumference to radius will differ since the value of pi remains the same while the radius changes.

For example, if we consider two circles, one with a radius of 2 cm and the other with a radius of 4 cm, the ratio of circumference to radius for the first circle will be 2π (since C = 2πr = 2π x 2 = 4π) and for the second circle, it will be 2π (since C = 2πr = 2π x 4 = 8π). Thus, the ratio of circumference to radius is not the same for every circle.

To know more about ratio visit:

https://brainly.com/question/12024093

#SPJ1

the likelihood of Erin getting at least one bullseye in 5 throws is 0.5563 or 55.63%.

To calculate the likelihood of Erin getting at least one bullseye, we need to first calculate the probability of her not getting a bullseye in a single throw. Since she scores a bullseye 15% of the time, the probability of her not getting a bullseye in a single throw is 85% (100% - 15%).

Using the probability of not getting a bullseye in a single throw, we can use the following formula to calculate the probability of not getting a bullseye in all 5 throws:

0.85 x 0.85 x 0.85 x 0.85 x 0.85 = 0.4437

Therefore, the probability of Erin not getting a bullseye in all 5 throws is 0.4437 or 44.37%.

To calculate the probability of Erin getting at least one bullseye in 5 throws, we can subtract the probability of her not getting a bullseye in all 5 throws from 1:

1 - 0.4437 = 0.5563

Therefore, the likelihood of Erin getting at least one bullseye in 5 throws is 0.5563 or 55.63%.

learn more about probability

https://brainly.com/question/30034780

#SPJ11

The probability that Erin will get at least one bullseye in her 5 throws at the adventure arcade is approximately 55.63%.

To find the probability that she will get at least one bullseye in 5 throws, we can use the complementary probability.

This means we will first find the probability of her not getting a bullseye in all 5 throws, and then subtract that from 1.

Find the probability of not getting a bullseye (1 - bullseye probability)

1 - 0.15 = 0.85

Calculate the probability of not getting a bullseye in all 5 throws

0.85^5 ≈ 0.4437

Find the complementary probability (probability of at least one bullseye)

1 - 0.4437 ≈ 0.5563

So, the probability that Erin will get at least one bullseye in her 5 throws at the adventure arcade is approximately 55.63%.

for such more question on probability

https://brainly.com/question/13604758

#SPJ11

To find the surface area of an object, we need to calculate the area of all its faces and add them up.

Assuming that "W" stands for the width, "l" for the length, and "h" for the height, the surface area of the object would be:

Area of the bottom face = length x width = 17' x 21' = 357 square feet

Area of the top face = same as the bottom face = 357 square feet

Area of the front and back faces = height x width = 19' x 17' = 323 square feet (each)

Area of the left and right faces = height x length = 19' x 21' = 399 square feet (each)

Therefore, the total surface area of the object would be:

357 + 357 + 323 + 323 + 399 + 399 = 2158 square feet.

So, the surface area of the object is 2158 square feet.