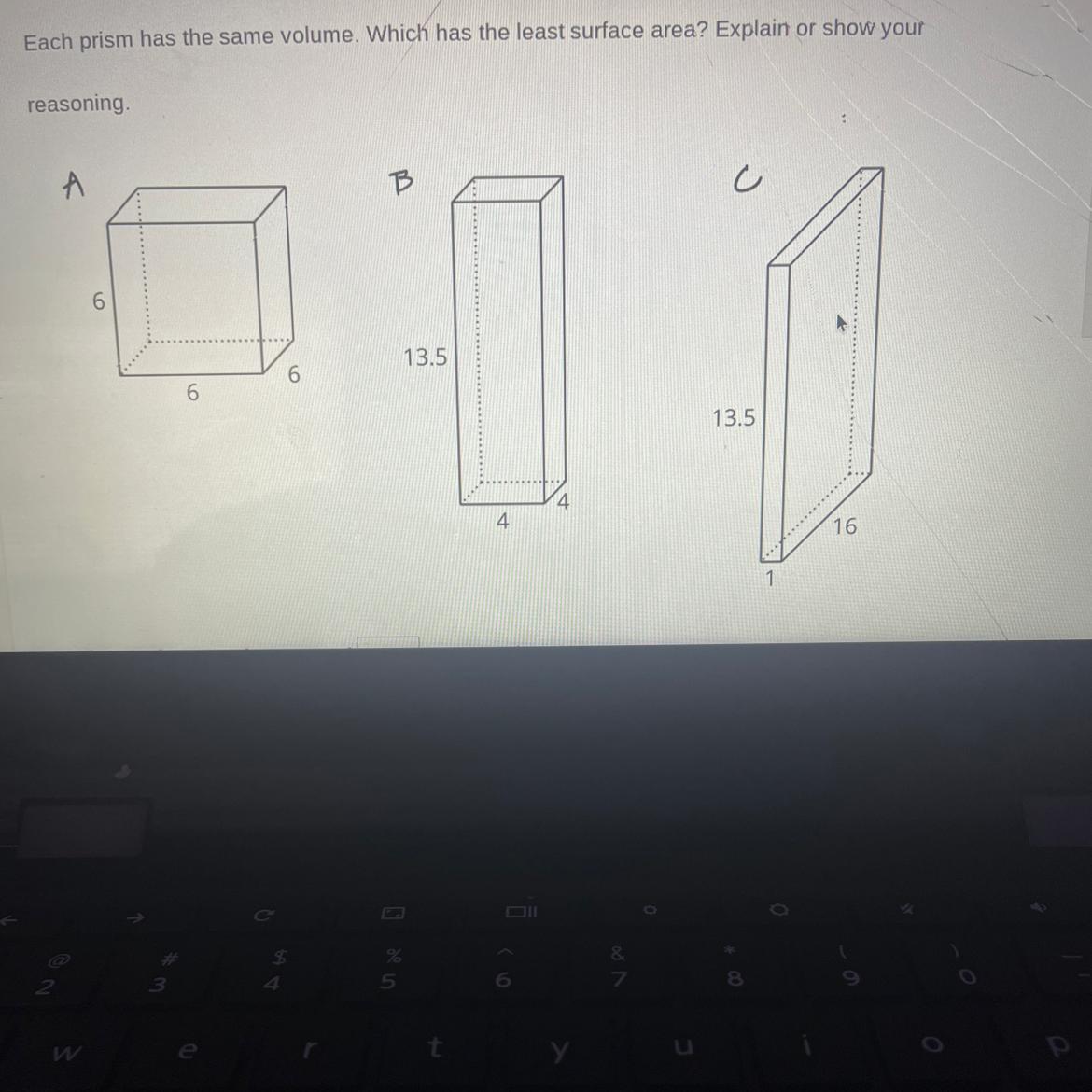
Each prism has the same volume. Which has the least surface area? Explain or show your
reasoning.

An exponential model for the worth of the savings account is [tex]W = 1250(1.045)^t[/tex]

The worth predicted by the exponential model is greater than predicted by the linear model at the end of 2020 by $131.2.

In Mathematics, an exponential function can be modeled by using the following mathematical equation:

[tex]f(x) = a(b)^x[/tex]

Where:

Based on the information provided about the savings account, we would determine the growth rate as follows;

[tex]W = P_{0}e^{rt}[/tex]

Growth rate, r = 1/(1 - 0)ln(1250/1306)

Growth rate, r = ln(1250/1306)

Growth rate, r = 0.0438

In the form [tex]W = a(b)^t[/tex], the required exponential function is given by;[tex]W = 1250(1.045)^t[/tex]

Years = 2020 -2010 = 10 years.

From the linear function, we have:

W = 56t + 1250

W = 56(10) + 1250

W = $1,810.

From the exponential function, we have:

[tex]W = 1250(1.045)^t\\\\W = 1250(1.045)^{10}[/tex]

W = $1,941.2

Difference = $1,941.2 - $1,810

Difference = $131.2.

Read more on exponential functions here: https://brainly.com/question/30241796

#SPJ1

The option that would result in the smallest margin of error in estimating the mean salt content, u, is 95% confidence, n = 50. The correct answer is Option D.

The margin of error is the amount by which a statistic is expected to differ from the true value of the population parameter. The interval estimate is calculated with the help of a margin of error. The margin of error and the interval estimate are inversely related to each other. If we want a small margin of error, we must increase the sample size.

The confidence level is the likelihood that a population parameter will fall within a specified range of values. The confidence level is determined by the sample size and margin of error. The sample size and margin of error are directly related to each other. When the sample size is smaller, the margin of error is larger. When the sample size is larger, the margin of error is smaller.

The margin of error is the highest at a confidence level of 50%. In general, as the confidence level increases, the margin of error decreases, and vice versa. As the sample size increases, the margin of error decreases. It follows that a 95% confidence level, n = 50 would yield the smallest margin of error in estimating the mean salt content, u. Hence, option D) 95% confidence, n = 50 would result in the smallest margin of error in estimating the mean salt content, u.

Learn more about margin of error here: https://brainly.com/question/29328438

#SPJ11

The speed of the particle is 8 m/s, the force of resistance is [tex]0.3(8)^2[/tex], or 19.2 N.

A particle of mass 1.2 kg is moving with a speed of 8 m/s in a straight line on a horizontal table. A resistance force is applied to the particle in the direction of the motion. The magnitude of the force is proportional to the square of the speed, such that [tex]F=0.3v^2[/tex]

The force of resistance is an opposing force that acts to reduce the speed of the particle. As the particle moves faster, the resistance force increases. The force is proportional to the square of the speed, meaning that if the speed doubles, the force is multiplied by four. The force is also in the same direction as the motion, meaning that it will reduce the speed of the particle.

The equation for the force of resistance is [tex]F=0.3v^2[/tex], where v is the speed of the particle. Therefore, if the speed of the particle is 8 m/s, the force of resistance is [tex]0.3(8)^2,[/tex] or 19.2 N. This means that the force of resistance acting on the particle is 19.2 N.

for such more question on resistance

https://brainly.com/question/28135236

#SPJ11

The missing dimension of the cone is its height, which is approximately 2.5 m when rounded to the nearest tenth.

We can use the formula for the volume of a cone, which is:

Volume = (1/3)πr²h

where r is the radius of the base and h is the height of the cone.

We are given the volume of the cone as 13.4 m³ and the radius as 3.2 m. Substituting these values into the formula, we get:

13.4 = (1/3)π(3.2)²h

Multiplying both sides by 3 and dividing by π(3.2)², we get:

h = 3 × 13.4 / π(3.2)²

h ≈ 2.5 m

Find more about volume of the cone

brainly.com/question/1578538

#SPJ4

BC will be congruent to AD under the C.P.C.T rule (corresponding parts of congruent triangles.)

Triangle congruence: Two triangles are said to be congruent if their three corresponding sides and their three corresponding angles are of identical size.

You can move, flip, twist, and turn these triangles to produce the same effect. When relocated, they are parallel to one another.

Two triangles are congruent if they satisfy all five conditions for congruence.

They include the right angle-hypotenuse-side (RAHS), angle-side-angle (ASA), angle-angle-side (AAS), side-side-side (SSS), and angle-side-angle (SSS) (RHS).

So, in the given △DAB and △DCB:

AC = AC = Common

∠DAC = ∠BAC = AC is the angle bisector

∠DCA = ∠BCA = AC is the angle bisector

Then, △DAB ≅ △DCB under the ASA congruency rule,

Then, BC will be congruent to AD under the C.P.C.T rule (corresponding parts of congruent triangles.)

Therefore, BC will be congruent to AD under the C.P.C.T rule (corresponding parts of congruent triangles.)

Know more about triangle congruency here:

https://brainly.com/question/2938476

#SPJ1

Answer:

i don't know i haven't done integers in a long time

Step-by-step explanation:

Alexis has 12 quarters and 8 dimes.

Let's use d to represent the number of dimes and q to represent the number of quarters. We know that Alexis has a total of 20 coins, so d + q = 20.

We also know that the value of these coins is $3.80. Since dimes are worth $0.10 and quarters are worth $0.25, we can write an equation for the total value in cents:

10d + 25q = 380

To make things easier, let's divide both sides of the equation by 5:

2d + 5q = 76

Now we can use the first equation to solve for d in terms of q:

d + q = 20

d = 20 - q

Substituting this into the second equation gives:

2(20 - q) + 5q = 76

Expanding the parentheses and simplifying, we get:

40 - 2q + 5q = 76

3q = 36

q = 12

Therefore, Alexis has 12 quarters. We can check this by plugging q back into the first equation to find that she has 8 dimes as well:

d + q = 20

d + 12 = 20

d = 8

The total value of 12 quarters and 8 dimes is:

12 quarters x $0.25 per quarter + 8 dimes x $0.10 per dime = $3.00 + $0.80 = $3.80


To know more about quarters and dimes refer here:

https://brainly.com/question/6500943#

#SPJ11

The measure of angle R in ΔSRT which is drawn inside the circle is 77.5°.

Circle is a two-dimensional shape that is defined as the set of all points that are equidistant from a central point. It is often represented as a round shape with a curved boundary.

Since SR is a diameter of the circle, it follows that angle STR is a right angle (90°). Therefore, we can find the measure of angle SRT using the following equation:

∠SRT + ∠STR = 180°

(2x-23°) + 90° = 180°

2x + 67° = 180°

2x = 180° - 67°

2x = 113°

x = 56.5°

∠TRS = 5x-97°

∠TRS = 5(56.5°)-97°

∠TRS = 192.5°

Finally, we can find the measure of angle SRT:

∠SRT = 180° - ∠STR - ∠TRS

∠SRT = 180° - 90° - 192.5°

∠SRT = -102.5°

Therefore, to find the measure of angle R, we need to add 180° to angle SRT:

∠R = ∠SRT + 180°

∠R = -102.5° + 180°

∠R = 77.5°

To know more about angle visit:

https://brainly.com/question/13483158

#SPJ1

If a sample of 50 items are chosen at random.  the probability that the sample mean length is greater than 9.1 inches is 0.0571, or 5.71%.

The sample mean of the 50 items follows a normal distribution with mean equal to the population mean (μ), and standard deviation  equal to σ/√n, where n is the sample size.

Substituting the given values, we have:

= μ = 9 inches

= σ/√n = 1/√50 inches

Now, we need to standardize the sample mean distribution to find the corresponding z-score using the formula:

z = (X- μX) / σX

Substituting the given values, we have:

z = (9.1 - 9) / (1/√50) = 1.58

The probability of getting a z-score of 1.58 or less is 0.9429. Therefore, the probability of getting a z-score of 1.58 or greater is:

P(z > 1.58) = 1 - P(z ≤ 1.58) = 1 - 0.9429 = 0.051

Therefore the probability that the sample mean length is greater than 9.1 inches is 0.0571.

Learn more about probability here:https://brainly.com/question/24756209

#SPJ1

Answer: 6

Step-by-step explanation:

The factors of 42 are: 1, 2, 3, 6, 7, 14, 21, 42

The factors of 78 are: 1, 2, 3, 6, 13, 26, 39, 78

Then the greatest common factor is 6.

Heres something you need to learn about the greatest common factor (gcf)

What is the Greatest Common Factor?

The largest number, which is the factor of two or more numbers is called the Greatest Common Factor (GCF). It is the largest number (factor) that divide them resulting in a Natural number. Once all the factors of the number are found, there are few factors that are common in both. The largest number that is found in the common factors is called the greatest common factor. The GCF is also known as the Highest Common Factor (HCF)

Let us consider the example given below:

Greatest Common Factor (GCF)

For example – The GCF of 18, 21 is 3. Because the factors of the number 18 and 21 are:

Factors of 18 = 2×9 =2×3×3

Factors of 21 = 3×7

Here, the number 3 is common in both the factors of numbers. Hence, the greatest common factor of 18 and 21 is 3.

Similarly, the GCF of 10, 15 and 25 is 5.

How to Find the Greatest Common Factor?

If we have to find out the GCF of two numbers, we will first list the prime factors of each number. The multiple of common factors of both the numbers results in GCF. If there are no common prime factors, the greatest common factor is 1.

Finding the GCF of a given number set can be easy. However, there are several steps need to be followed to get the correct GCF. In order to find the greatest common factor of two given numbers, you need to find all the factors of both the numbers and then identify the common factors.

Find out the GCF of 18 and 24

Prime factors of 18 – 2×3×3

Prime factors of 24 –2×2×2×3

They have factors 2 and 3 in common so, thus G.C.F of 18 and 24 is 2×3 = 6

Also, try: GCF calculator

GCF and LCM

Greatest Common Factor of two or more numbers is defined as the largest number that is a factor of all the numbers.

Least Common Multiple of two or more numbers is the smallest number (non-zero) that is a multiple of all the numbers.

Factoring Greatest Common Factor

Factor method is used to list out all the prime factors, and you can easily find out the LCM and GCF. Factors are usually the numbers that we multiply together to get another number.

Example- Factors of 12 are 1,2,3,4,6 and 12 because 2×6 =12, 4×3 = 12 or 1×12 = 12. After finding out the factors of two numbers, we need to circle all the numbers that appear in both the list.

Greatest Common Factor Examples

Example 1:

Find the greatest common factor of 18 and 24.

Solution:

First list all the factors of the given numbers.

Factors of 18 = 1, 2, 3, 6, 9 and 18

Factors of 24 = 1, 2, 3, 4, 6, 8, 12 and 24

The largest common factor of 18 and 24 is 6.

Thus G.C.F. is 6.

Example 2:

Find the GCF of 8, 18, 28 and 48.

Solution:

Factors are as follows-

Factors of 8 = 1, 2, 4, 8

Factors of 18 = 1, 2, 3, 6, 9, 18

Factors of 28 = 1, 2, 4, 7, 14, 28

Factors of 48 = 1, 2, 3, 4, 6, 8, 12, 16, 24, 48

The largest common factor of 8, 18, 28, 48 is 2. Because the factors 1 and 2 are found all the factors of numbers. Among these two numbers, the number 2 is the largest numbers. Hence, the GCF of these numbers is 2.

If you know it, dont read it

The probability of selecting two non-defective DVD players from a group of six is 2/5. This is based on the assumption that the selection is done without replacement.

We can use the formula for calculating probabilities of combinations:

P(not defective) = number of ways to choose 2 non-defective DVD players / total number of ways to choose 2 DVD players

Total number of ways to choose 2 DVD players out of 6 is:

C(6,2) = 6! / ([2!] [4!]) = 15

Number of ways to choose 2 non-defective DVD players out of 4 is:

C(4,2) = 4! / ([2!] [2!]) = 6

Therefore, the probability that Cecil chooses 2 non-defective DVD players is:

P(not defective) = 6/15 = 2/5

So the value of P(not defective) is 2/5.

Learn more about probability here: brainly.com/question/30034780

#SPJ4

The best type of inspection to use depends on the nature of the purchase. Each type of inspection has its own advantages and disadvantages, and should be chosen based on the requirements of the product and the level of risk associated with the inspection.

See more about purchase at: https://brainly.com/question/27975123

#SPJ11

Answer:

The answer should be 20,365.714 but I am not sure

Step-by-step explanation:

The measure of the larger acute angle of the triangle can be calculated using trigonometric ratios or by subtracting the measure of the smaller acute angle from 90 degrees. Without further information or given measurements, it is not possible to determine the exact measure of the angle.

Let's consider the general formula for a right triangle where A, B, and C are the angles and a, b, and c are the corresponding sides opposite to each angle:

sin A = a/c, sin B = b/c, and sin C = a/b.

For an acute triangle, we know that the sum of all the angles is equal to 180 degrees, so A + B + C = 180. If the triangle is a right triangle, then one of the angles, say C, is equal to 90 degrees, and A + B = 90 degrees.

In this case, we are only given that the angles of the triangle are acute. Therefore, we can use the formula sin A = a/c, sin B = b/c and sin C = a/b to solve for the angles or use the fact that A + B + C = 180 degrees and A + B = 90 degrees to find the measure of the larger acute angle by subtracting the measure of the smaller acute angle from 90 degrees. However, without specific measurements or additional information, we cannot determine the exact measure of the angle.

for such more question on triangle

https://brainly.com/question/1058720

#SPJ11

The perimeter of the updated blueprint will be 24 times the sum of the original length and width.

If Ezra wants to multiply the dimensions of the stage by a certain percentage to make the image 12 times larger than the original, he needs to find out what percentage that is.

To do this, he can divide the desired size of the new stage by the original size of the stage, and then multiply by 100 to get the percentage increase. So, if the original blueprint dimensions are x by y, and he wants to make the image 12 times larger, the new dimensions will be 12x by 12y.

To find the percentage increase, he can use the following formula:

Percentage increase = [(new size - original size) / original size] x 100

In this case, the new size is 12 times the original size, so the formula becomes:

Percentage increase = [(12x * 12y - x * y) / (x * y)] x 100

Simplifying this expression gives:

Percentage increase = [(144xy - x * y) / (x * y)] x 100 = 14300%

Therefore, Ezra needs to multiply the dimensions of the stage by 14300% to make the image 12 times larger than the original blueprint.

To find the perimeter of the updated blueprint, he can use the formula for the perimeter of a rectangle, which is: Perimeter = 2(length + width)

In this case, the length and width have been multiplied by 12, so the new perimeter becomes:

Perimeter = 2(12x + 12y) = 24(x + y)

Therefore, the perimeter of the updated blueprint will be 24 times the sum of the original length and width.

To learn more about perimeter here:

brainly.com/question/6465134#

#SPJ11

The probability that a randomly selected adult weighs between 120 and 165 lbs is approximately 0.8186.

Since the weights of adults are normally distributed with a mean of 140 lbs and a standard deviation of 25 lbs, we can use the standard normal distribution to calculate the probability.

We first need to standardize the values using the formula: z = (x - μ) / σ, where x is the weight, μ is the mean, and σ is the standard deviation.

For x = 120 lbs, z = (120 - 140) / 25 = -0.8, and for x = 165 lbs, z = (165 - 140) / 25 = 1.0. We can then use a calculator to find the probability between -0.8 and 1.0, which is approximately 0.8186.

Thus, the chance of picking an adult at random who weighs between 120 and 165 lbs is roughly 0.8186.

To know more about normally distributed refer here:

https://brainly.com/question/29509087#

#SPJ11

The total number of hours is 30 hours as the sum of 7/8 and 3/8 comes out to be 30 hours.

1) We know that there is a total of 24 hours in a day.

therefore, 7/8 hours of Wednesday =

number of hours in a day = 24

number of hours every Wednesday = 7/8

= 7/8 x 24 hours

= 7 x 3 hours

= 21 hours

7/8 hours every Wednesday means 21 hours every Wednesday.  

2) We know that there are a total of 24 hours in a day;

therefore, 3/8 hours of Friday =

number of hours in a day = 24

number of hours every Friday = 3/8

= 3/8 x 24 hours

= 3 x 3 hours

= 9 hours

3/8 hours every Friday means 9 hours every Friday.

therefore, the total number of hours = 21 + 9 = 30

The total number of hours is 30 hours as the sum of 7/8 and 3/8 comes out to be 30 hours.

To learn more about sum, click here:

brainly.com/question/29034036

#SPJ4

The 4 in the hundredths place has a smaller value than the 4 in the tens place.

In the decimal number system, each digit to the left of the decimal point represents a power of 10, starting with 10^0 = 1 for the rightmost digit. Each digit to the right of the decimal point represents a negative power of 10, with the place value decreasing as you move farther to the right.

In the number 240.149, the 4 in the tens place represents 4 x 10 = 40. The 4 in the hundredth place represents 4/100 or 0.04, which is smaller than 40. Therefore, the 4 in the tens place has a greater value than the 4 in the hundredths place.

Hence, the value of a digit in a decimal number depends on its position relative to the decimal point. Digits to the left of the decimal point represent whole numbers, while digits to the right of the decimal point represent fractions or parts of a whole.


To know more about the decimal number system, refer here:

https://brainly.com/question/30477589#

#SPJ11

A causal study is a study that seeks to determine whether one variable causes another variable.

The independent variable is the variable that is believed to cause the change in the dependent variable, while the dependent variable is the variable that is believed to be influenced by the independent variable.

In a causal study of the effect of shelf placement on sales of a brand of cereal, the independent variable is where the cereal was placed on the shelf. The dependent variable is sales of the cereal.

This is because the sales of the cereal are influenced by where it is placed on the shelf.The answer to the question is sales of the cereal.

To know more about independent variable click on below link:

https://brainly.com/question/1479694#

#SPJ11

There are a total of 32 different possible footwear choices that we can make.

Given, The number of pairs of socks = 4

The number of pairs of shoes = 4

We are to find out the number of possible footwear choices we can make if we can wear any combination of socks and shoes, including mismatched pairs.

So, We can wear any pair of socks with any pair of shoes including a mismatch.

Thus, for each pair of socks, there are 4 possible pairs of shoes.

And for each pair of shoes, there are 4 possible pairs of socks.

Therefore, we can form,

Total number of possible footwear choices = 4 pairs of socks * 4 pairs of shoes * 2 (considering the case of mismatched pairs) = 32 pairs.

For similar question on combination.

https://brainly.com/question/24306044

#SPJ11

Answer:

Step-by-step explanation:

a. 100 divided by 75 = 1.3333333333333333333333333333333

1.3333333333333333333333333333333 times 43 = 57.333333333333333333333333333332

round it to the nearest whole number: ≅ 57%

So, the system of equations solution is (x, y) = (-27/8, 31/4), and the value of y in this solution is 31/4, which is roughly 7.75. As a result, the answer is y = 31/4.

An equation is a statement in mathematics that states the equality of two expressions. An equation has two sides that are separated by an algebraic equation (=). For example, the argument "2x + 3 = 9" asserts that the phrase "2x + 3" equals the value "9". The purpose of equation solving is to determine which variable(s) must be changed in order for the equation to be true. Simple or complex equations, regular or nonlinear equations, and equations with one or more elements are all possible. In the equation "x2 + 2x - 3 = 0," for example, the variable x is raised to the second power. Lines are employed in a variety of mathematical disciplines, including algebra, calculus, and geometry.

the system of equations,

[tex]y = 1 - 2x\\2x - 3(1 - 2x) = -30\\2x - 3 + 6x = -30\\8x = -27\\x = -27/8\\2(-27/8) + y = 1\\-27/4 + y = 1\\y = 1 + 27/4\\y = 31/4[/tex]

So, the system of equations solution is (x, y) = (-27/8, 31/4), and the value of y in this solution is 31/4, which is roughly 7.75.

As a result, the answer is y = 31/4.

To know more about equation visit:

https://brainly.com/question/649785

#SPJ1

Answer: No

Step-by-step explanation:

Because the length of the box is shorter than the length of the club

20in<26in

The width of the box is also shorter than the width of the club

13in<16in

The height of the box is also shorter than the height of the club

11in<16in

But what about putting it at an angle?

So we know  [tex]a^{2} +b^{2} =c^{2}[/tex]

so let's try [tex]20^{2} +13^{2} =x^{2}[/tex]

                               [tex]x^{2}[/tex]=569

                               [tex]x=\sqrt{159}[/tex]

x is near 23.85 in, but 23.85<26. So no.

The sum of the first 50 terms is 3775. Let a be the first term and d be the common difference of the arithmetic sequence.

Then, the tenth term is a + 9d = 28, and the sum of the fifth and seventh terms is 2a + 12d = 32.

Solving these equations simultaneously, we get a = 2 and d = 3.

To find the sum of the first 50 terms, we use the formula for the sum of an arithmetic sequence:

S50 = (50/2)(2a + (50-1)d) = 25(2 + 49(3)) = 3775.

Find out more about arithmetic sequence

brainly.com/question/15412619

#SPJ4

Answer:

Step-by-step explanation:

The formula for the volume of a cylinder is:

V = πr^2h

where V is the volume, r is the radius, and h is the height.

We are given that V = 1607 and r = 4. We can plug these values into the formula and solve for h:

1607 = π(4^2)h

1607 = 16πh

h = 1607/(16π)

h ≈ 25.5

Therefore, the height of the cylinder is approximately 25.5 units. Note that we rounded the answer to one decimal place since the radius was given to one decimal place.

The bottom of the ladder is moving at a rate of 4/3 ft per second.

To solve the problem, we can use the Pythagorean Theorem:[tex]$x^2 + y^2 = 64$[/tex], where x is the distance from the wall to the bottom of the ladder and y is the length of the ladder. We differentiate this equation with respect to time t and use the chain rule to get [tex]$\frac{d}{dt} (x^2 + y^2) = \frac{d}{dt} 64$[/tex]

Simplifying, we get

[tex]$2x \frac{dx}{dt} + 2y \frac{dy}{dt} = 0$[/tex]

When the bottom of the ladder is 4 feet from the wall, we have x = 4 and y = 8, so we can substitute these values into our equation and solve for [tex]$\frac{dx}{dt}$[/tex]:

[tex]$2(4)\frac{dx}{dt} + 2(8)(-2) = 0$[/tex]

[tex]$\frac{dx}{dt} = \frac{16}{8} = \frac{4}{3}$[/tex]

Therefore, the bottom of the ladder is moving at a rate of [tex]$\frac{4}{3}$[/tex] ft/s.

Learn more about Time:

https://brainly.com/question/11733843
#SPJ4

The longest side of a triangle with angles of 50°, 60°, and 70° and a length of 9 furlongs on the shortest side is approximately 12.2 furlongs.

             The Law of Cosines is used to find the remaining parts of an oblique (non-right) triangle when either the lengths of two sides and the measure of the included angle are known (SAS) or the lengths of the three sides (SSS) are known.

         To calculate this, using the Law of Cosines formula,

  which is:

                [tex]c^2 = a^2 + b^2 - 2abcosC[/tex]

where c is the longest side, a is the shortest side, b is the other side of the triangle, and C is the angle

between a and b.

In this case, c = 12.2 furlongs,

                    a = 9 furlongs,

                    b is the side opposite the angle 70°, and C = 70°.

So the formula becomes:

        [tex]c^2 = 92 + b^2 - 2(9)(b)cos70^{o}[/tex]

Solving for b gives us b = 12.2 furlongs, which is the length of the longest side.

Learn more about triangles with the angle and length, of the longest side at: https://brainly.com/question/11011280

#SPJ11

Coupling tetraalkylammonium and ethylene glycol ether side chain is an effective method to enable highly soluble anthraquinone-based ionic species for nonaqueous redox flow battery. The side chains of the tetraalkylammonium are modified with the ethylene glycol ether, which is a highly polar solvent, allowing for better solubility in nonaqueous electrolyte solutions. Additionally, the ethylene glycol ether has the ability to modify the stability of the ionic species, preventing aggregation and ensuring the longevity of the battery. This increases the redox capacity and enhances the performance of the flow battery.

The ethylene glycol ether-tetraalkylammonium coupling has been proven to be an effective method for improving the solubility and stability of anthraquinone-based ionic species. For example, it has been observed that the coupling of ethylene glycol ether to anthraquinone-based ionic species enhanced the current density of the battery by more than 3 times. Furthermore, the coupling process has also been found to improve the energy efficiency and storage capacity of the nonaqueous redox flow battery.

Overall, coupling tetraalkylammonium and ethylene glycol ether side chain is an effective method for enabling highly soluble anthraquinone-based ionic species for nonaqueous redox flow battery. This process has been proven to improve the performance of the battery, including current density, energy efficiency, and storage capacity.

for such more questions on solubility

https://brainly.com/question/23946616

#SPJ11

There are 5²⁵ possible ways to select 25 cans of soda from 5 types, while there are [5¹⁸] (25 choose 7) possible ways to select 25 cans with at least 7 Dr. Peppers, and only [3²²] (25 choose 3) possible ways to select 25 cans with only 3 Dr. Peppers available.

(a) Since there are five types of soda and we are selecting 25 cans, we can choose any type of soda for each can. Therefore, the number of ways to select 25 cans of soda is 5²⁵.

(b) If we must include at least seven Dr. Peppers, then we can choose the remaining 18 cans from any of the five types of soda (including Dr. Pepper). We can choose 7 Dr. Peppers in (25 choose 7) ways. Therefore, the number of ways to select 25 cans of soda with at least seven Dr. Peppers is (25 choose 7) [5¹⁸].

(c) If there are only three Dr. Peppers available, then we must choose all three Dr. Peppers and select the remaining 22 cans from the four types of soda (excluding Dr. Pepper). We can choose the remaining 22 cans in 4²² ways. Therefore, the number of ways to select 25 cans of soda with only three Dr. Peppers available is 3 [4²²].

Learn more about Combinatorics here: brainly.com/question/13261685

#SPJ4

Complete question:

From an unlimited selection of five types of soda, one of which is Dr. Pepper, you are putting 25 cans on a table.

(a) Determine the number of ways you can select 25 cans of soda.

(b) Determine the number of ways you can select 25 cans of soda if you must include at least seven Dr. Peppers.

(c) Determine the number of ways you can select 25 cans of soda if it turns out there are only three Dr. Peppers available.